vasupworlds.blogg.se - How to use symbolic math toolbox in matlab

#How to use symbolic math toolbox in matlab pdf#
#How to use symbolic math toolbox in matlab manual#
#How to use symbolic math toolbox in matlab verification#

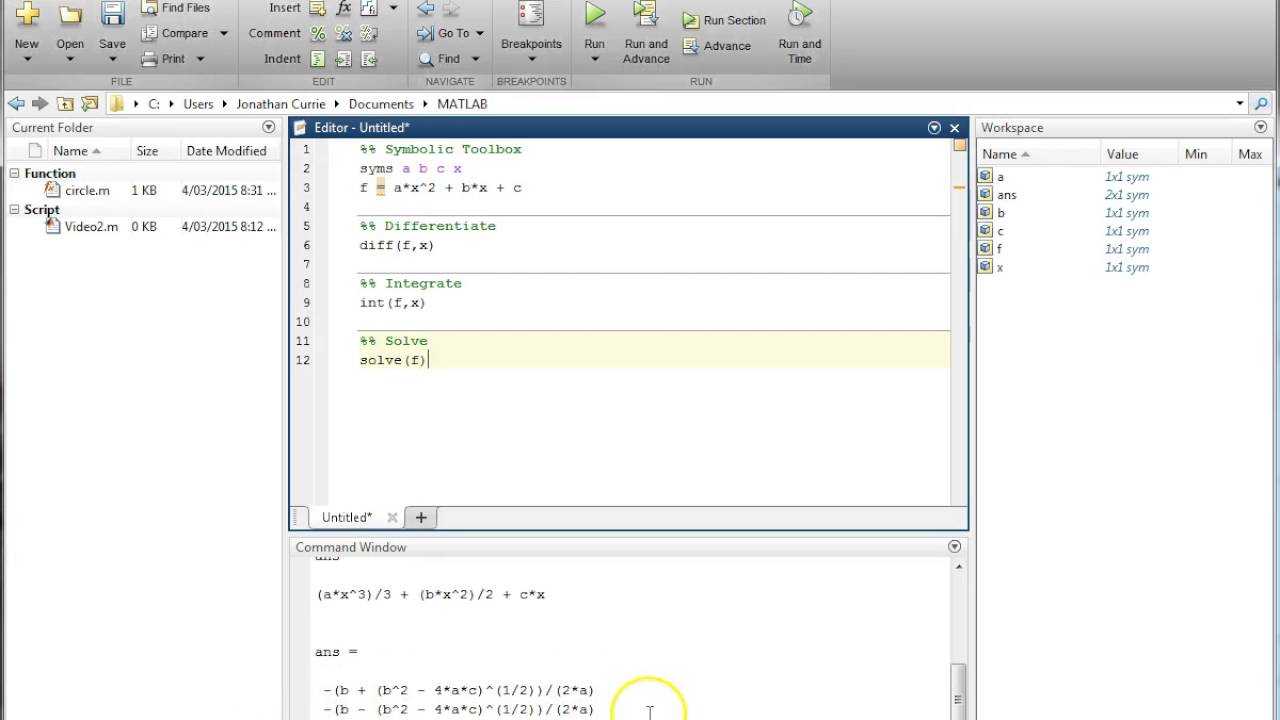
Now we can easily solve the original initial value problem by typing f(t) = dsolve(besselODE, y(1)=1, y(2)=n) Note that we used diff to introduce the symbolic derivatives of y and the = sign to set up a symbolic equation. Now we define the Bessel ODE by typing besselODE = t^2*diff(y,2) + t*diff(y) + (t^2-n^2)*y = 0 Now the dependent ODE variable is declared as a symfun (symbolic function) y(t): syms n y(t) Īfter executing the last command, we can see the symfun y as well as the symbolic variables n and t in the MATLAB workspace. Improving Our Workflow Using Symbolic Functions And Symbolic Equationsīefore starting with any new computations, let us clean up the workspace: clear all These new features let you make the worklfow for solving ODEs and testing solutions much more smooth and convenient. In R2012a, symbolic equations and symbolic functions were introduced in the Symbolic Math Toolbox.

#How to use symbolic math toolbox in matlab verification#

Verification of solutions and initial conditions is not convenient, since we cannot use the subs command on string representations of ODEs.

When using variables inside a string and afterwards assigning values to these variables, the values do not show up in the string.

#How to use symbolic math toolbox in matlab manual#

Manual work has been required to overcome these limitations of string syntax: But our workflow was not completely smooth. We have solved the ODE, and we have checked the correctness of the solutions. Do f(1) = 1 and f(2) = n really hold? checkInitCond1 = subs(f,t,1) Result = simplify(t^2*diff(f,2)+t*diff(f)+(t^2-n^2)*f)įinally, we should also check the initial values. But because the string input does not let us use subs to directly plug the solution into the ODE, we, again, have to do some manual work: syms t In order to verify that the solution is correct, we need to plug it into the ODE and see if this gives 0. (bessely(1, 1) - bessely(1, 2)) besselj(1, t)Īnother limitation becomes obvious when we try to check the solution. We more or less have to modify the ODE by hand replacing all appearances of n by 1: f = dsolve( 't^2*D2y+t*Dy+(t^2-1^2)*y', 'y(1)=1', 'y(2)=1', 't') Just defining n = 1Īnd re-evaluating the command f = dsolve(besselODE, 'y(1)=1', 'y(2)=n', 't') ĭoes not work, since the value for n shows up in the string defining the differential equation: besselODE Say you want to assign a special value to n, e.g., n = 1, and solve the equation again using this new value for n. Such string-based input for the dsolve function has some limitations. (n bessely(n, 1) - bessely(n, 2)) besselj(n, t) (n besselj(n, 1) - besselj(n, 2)) bessely(n, t)īesselj(n, 1) bessely(n, 2) - besselj(n, 2) bessely(n, 1) Here is a typical example that shows how you can solve a Bessel ODE with two given initial values: besselODE = 't^2*D2y+t*Dy+(t^2-n^2)*y' į = dsolve(besselODE, 'y(1)=1', 'y(2)=n', 't') The usual way to solve ordinary differential equations (ODEs) using the Symbolic Math Toolbox dsolve command is to set up the equations using string syntax. Solving Ordinary Differential Equations Using String Input

Then we compute the same results again using symbolic equations and symbolic functions and discuss how this improves our workflow.

First, we solve the differential equation using string syntax and point out some limitations of string syntax.

If you have done programming in any other high-level programming language like C, C++ or Java, then it will be very much beneficial and learning MATLAB will be like a fun for you. We assume you have a little knowledge of any computer programming and understand concepts like variables, constants, expression, statements, etc. After completing this tutorial you will find yourself at a moderate level of expertise in using MATLAB from where you can take yourself to next levels.

$how to use symbolic math toolbox in matlab$

This tutorial has been prepared for the beginners to help them understand basic to advanced functionality of MATLAB. Problem-based MATLAB examples have been given in simple and easy way to make your learning fast and effective. It is designed to give students fluency in MATLAB programming language. This tutorial gives you aggressively a gentle introduction of MATLAB programming language. It can be run both under interactive sessions and as a batch job. It started out as a matrix programming language where linear algebra programming was simple. MATLAB is a programming language developed by MathWorks.

#How to use symbolic math toolbox in matlab pdf#

PDF Version Quick Guide Resources Job Search Discussion